

The discovery of π (Pi) in the Great Pyramid is the most famous pyramid riddles at all. Even people who never heard about “numerology” know about it. And it is one of the oldest pyramid riddles at all, published in 1854 and until today the centre of many publications about pyramid mysteries.
Let us have a look at the original π-definition of John Taylor. He published it in 1854[1 ], and it was proven in 1864 by the Scottish astronomer Piazzi Smyth. They came more or less intuitively to the conclusion, that
|
|
| Fig. 1 - π-Definition |
This value would be really fantastic precise. There is only one problem: the estimation of the pyramid dimensions of Taylor and Smyth were overturned by reality more than 120 years ago. The measurements done by Flinders Petrie the “father of Egyptology”, which were checked and confirmed with minimal differences several times, show, that the pyramid base length is about 2.5 m shorter, and that it is 1.6 m lower as estimated. With the real measurements – base length 230,36 meter, height 146,59 meter – the results are not that spectacular, the modern π-value of 3,142916 has an error on the 3rd decimal. But Erich von Däniken even gave 1989 the at that time for almost 110 years disproved π-value of 3.1416 [2 ] - the clocks in the ancient alien genre are running much, much slower than in the rest of the world. And again a tip for EvD: the investment in a pocket calculator, with which the verification of such values is quick and easy possible, can be very useful :-)
A π-value with this precision is nothing special. My bureau has a width of 3.95 m and a height of 2.51 m at the door. If I use the pi-formula from the pyramid, I get a result of 3,1474, nearly as precise as in the Great Pyramid. Try it for yourself, measure some dimensions of buildings of your daily life and see, what “secrets” the builder have encoded there. Surely, the secret brotherhood of pyramid builders is still around :-)
|
|
| Fig. 2 -π-Definition |
π in itself is not a mysterious or even magical construct. π is simply the value you have to multiply the diameter of a circle with to get its circumference. The value is about 3.14149... and everyone working with round objects like wells and columns has inevitably something to do with π, even if he doesn't know it.
The rough value of π can be obtained through simple experiments. You take a wheel of 1 unit (meter, yard, cubit...) diameter, roll it on the ground for a complete revolution and measure then the distance it has gone on the ground. No secret, it will be something around 3.14 units. Or you can wrap a rope around a wheel and measure its length.
But we know that the old Egyptians never used that value for circular calculations. Even long after the pyramid time they used another “value” for π as we can see from the Mathematical Papyrus Rhind (MPR) from the Middle Kingdom. They used the approximation 256/81 which gave a value of 3.1605.[3 ] This is precise enough for every-day applications.
Above I wrote “value” in quotes, and this has a good reason. The real intellectual achievement is not finding out the value of π, but finding out the principle to use one constant for many different calculations having to do with circles or spheres. The circumference of a circle for example is 2 π * radius, and the area is π * radius2. To use this constant for many different calculations is what I call π-principle.
And exactly this was unknown in ancient Egypt. There are three lessons in the MPR dealing with circle calculations. One (lesson 43) calculates the volume of a cylindrical silo, lesson 48 compares the area of a square with the side length of x to a circle with the diameter of x, and lesson 50 describes how the Egyptians calculated the area of a circle. This one is most revealing, so I discuss it here. It uses a circle with a diameter of nine units:
"Take away 1/9 of the diameter. This is one here.
The remain is 8;
Multiply 8 by 8;
This is 64;
Therefore he (the circle, FD) contains 64 Setat of land"[4 ]
The other circle calculations also use this algorithm, which consists of three fundamental parts (divide diameter by nine, multiply result by eight and multiply the result with itself) and give exemplary results for different diameters.
This clearly shows, that the Egyptians knew nothing about the principle of π, to use one constant for all different circle diameters.
The “value” for the Egyptian π given in literature is only the value one gets if using the algorithm on a one-unit-circle and using the resulting value – a way the Egyptians never went.
|
| Fig. 3 - MPR lesson 48 |
The writer Ahmose drew a little sketch alongside of lesson 48 of the MPR which shows, what the Egyptians thought about the correlation between a circle and a square:[5 ]
If one divides each side of a square into nine parts, and cuts away the edges in a diagonal running through a 2 by 3 square, the result is an octagon with an area almost as large as an inscribed circle which touches the sides of the square.
This also demonstrates, that the Egyptians had no clue about the much more simple method of using a simple multiplication with the constant π.
By the way, there has not a single lesson about calculating the circumference of a circle been found yet. With the sketch of Ahmose this would have been relatively simple,
Those Egyptian methods were precise enough for home use, the difference to the “real” π was around one percent. For higher mathematics it was not usable at all.
The comparison between the theory of circle calculations and the methods used by the Egyptians leave us in a dilemma. Some find π where it makes no sense (in buildings with a square base), whereas we find no trace of it where it really matters, in their circle calculations. To say it with Erich von Däniken: Something doesn't fit here.
There are three different schools of thought which are affected in different ways:
But even the fans of a godly or alien pyramid have problems, because the Great Pyramid is not the only one with π in it. All together 10 large pyramids built between the 4th and 12th dynasty, which could be reconstructed so far, contain this constant. OK, the explanations for the later pyramids (the last one is the pyramid of Amenemhet III from the 12th Dynasty, almost 1000 years after Khufu) is easy – they are simple copies and the builders didn't know the secret. But if all other pyramids are copies, why don't they all contain π?
An even larger problem: Why does the first real pyramid, built in Meidum long before Khufu's, has π in it? (base length 275 cubits, height 175 cubits, π-value 3.1429) Therefore constant can't be a sign of God or of small furry animals...
“Siimple” I hear the friends of the first two π-schools say “the pyramid of Khufu is the oldest one and was built millennia before the other pyramids.” Well, this cannot be true as we saw on the previous pages.
However, coincidence as explanation for π in several pyramids is unsatisfactory. Is there perhaps a secret reason, why the Egyptians chose inclinations that lead to a π-approximation?
Pi in the pyramid made many so called rationalists nervous. They wanted an explanation without God, Atlanteans or small furry animals. They found a solution - that makes more problems than it can solve: the cubit drum[6 ]. The Egyptians, so the idea, measured long distances not with rope measures, but with drums. These drums hat one cubit in diameter and were rolled along, and the Egyptians counted the rotations, calculating the length. The height was measured by stacking the drums (or using cubit rods) – and so they got π inevitably into the pyramids
Unfortunately, the Egyptians were called “rope slingers” by their neighbours, and not “drum rollers” - because they measured large areas with rope triangles. Also, there is not a single piece of evidence in Egypt, that they ever used drums for measurements, on the contrary, many calculations were found in Egypt, using ropes as device. And the drum itself enlarges the problems.
It was so nice to see in a film. A drum was rolled three times on a floor, then three of them were stacked, and voila they had pi. But this would mean, that the Egyptians were so stupid not to notice, that the circumference of a drum is much larger than its diameter. This is so outrageous stupid that is a real slander against this old and sophisticated culture.
If the builders knew that the diameter if their drum was much smaller than its circumference (and they perhaps used their pi to calculate it) the appearance of this constant in the pyramid must have been planned right from the beginning. There is no other possibility (although they planned a 3.16 and got a 3.14).
Had the Egyptians used cubit drums to measure their buildings, Egypt must be full of strange unfinished buildings. No planning would have worked with their π-calculation Per revolution of the drum the difference between planned and measured length would differ about a centimetre. On the base length of the Great Pyramid, which needed for its 440 cubit about 139 ¾ revolutions, the error would sum up to about 1.40 meters. OK, if they had measured all distance with drums that would have gone unnoticed, but the inventors of that thesis argued, that we have no trace of the usage of measure drums because most measurements were made with ropes and rods, and that only long distance measurements were done by drum.
But that would have lead to collisions everywhere, where drum- and rope-measured dimensions came together. For example where the rope-measured mortuary temple and the drum-measured temenos(pyramid-surrounding)-wall met. On the length of the surrounding wall the error due to the wrong pi-value would had added up to about 6 meter or 11 cubits. Something the Egyptians would have noticed. The usage uf this method parallel to rope or rod measurements would inevitably have lead to finding a more precise value of π.
Also, the precision of those drums must have been phenomenal. If a measuring rod of 100 cubits had an error of 1 mm, the error would sum up to about 4 ½ millimetres over the length of the pyramid base length. If the drum had a 1 mm error, the error would have been 44 centimetres, 100 times larger, because the revolutions multiply the error. And what about degeneration and deformation of the drum? No, cubit rods were the better way to measure distance. They are not only documented, they even got their own name as a length unit. 100 cubits were called a “chet”, a rod.
Furthermore, if π gets inevitably into a pyramid with this measurement method, why no not all pyramids contain π? The two neighbouring pyramids of Khufu have a “π-value” of 3.00 (Kafre) and 3.26 (Menkaure) – no trace of π. Here the inventors of the cubit drum have the same problems as the copy-friends of the God- and alien-pyramids. No, the cubit drum is no solution.
|
|
| Fig. 4 Angle |
There is a relatively easy solution based on the unit system the Egyptians used. After we have examined it everyone will ask the question, why there ever was something like a π-mystery!
The usage of “angles” is so fundamental in our culture, that many can not think about another method of measuring inclinations. Measuring angles is reading up the difference between two intersecting lines on a circle divided in 360 parts, with the intersection of the lines in the centre of the circle. This method was invented in Mesopotamia – and never found its way into ancient Egypt!
Many other cultures, as the Egyptians, measured slopes. They measured, how many cubits run were necessary for one cubit rise. Or hands, or fingers, or combined. The method is used today for example in road signs, a slope of 18% means a rise of 18 meters lies on a distance of 100 meters. This would be an angle of about 10°.
Additionally, the Egyptians had some unit conversion ant mathematical constraints:
Fortunately, the Egyptians were so kind to leave us some calculations of triangles (MPR lessons 51, 52) and even pyramid stumps (Papyrus Moscow lesson 28)[7 ]. From them we see that they used preferably right angled triangles with whole cubit values on the base side and the height. And normally in the form “to the length of x cubits is a height of n cubits”- slopes were always given as “n units length to one unit height”.
The pyramid also is a slope, but one that is steeper than one that can be constructed this way – the steepest one is 0ne unit rise to one unit run = 45°. Therefore for the run the next smaller unit must be used, hands or even finger run to the cubit height. And, lo, 22 fingers run to the cubit rise gives exactly the inclination Khufu's pyramid was built with. The pyramid of Khafre, right beside, was built exactly with a 21 fingers run to 1 cubit-slope.
With this method, exactly 28 angles with whole finger units can be constructed, from 1 finger to the cubit (2.04°) to 28 fingers to the cubit (45°). Naturally, shallower slopes were constructed. The descending passages in the pyramids are constructed all in a slope of 45 to 56 fingers per cubit, and slopes using a run to two cubits height are known
The clou: a slope of 22 fingers to the cubit gives with Taylor's π-formula “double base length (= 4 slope base lengths) divided by height exactly 88/28 fingers = 3.142857. This is a π-approximation, although not known by the builders. If we leave aside the idea that the Egyptians purposefully constructed their measurement systen so that a 22:1 slope gives π. This we leave with the conspiracy theorists :-)
Uh oh, again I was overtaken by reality. In a discussion on the Maat-Messageboard, where some pyramid researchers tried to prove that the Egyptians knew π, one author claimed, that not the Great Pyramid was planned in the cubit/hand/finger-system, but that this measurement system was invented by measuring the age old, π containing pyramid, and therefore bringing π into it..
Let's put aside this bizarre idea. If we can demonstrate, that the pyramid is built nearer to the 22:1 slope than to π, we have a strong argument. Let's check:
The Great Pyramid has a “π-value” of 3.142916, the slope of 22:1 gives a value of 3.142857, the difference is only 0.000059.
π = 3-141593 , the difference to the pyramid value is 0.00132364. Therefore the pyramid is built 20 times more precise to the 22:1 slope than to π!
That leaves the question, why they used that slope. Did the builder possibly knew, that this slope was near to π and used it therefore?
Not really. Although we do not know the symbolism behind the pyramid form, all those monuments since the first step mastaba were built with a hull inclination between 45° and 55°[8 ]. This equal slopes between 20:1 and 28:1, and we can see a trend to steeper buildings. The first large pyramid form, the step mastaba of Zoser, had a hull slope of 25:1, the shallowest one with the exception of the Red Pyramid in Dahshur. The later step pyramids were planned with a 24:1 hull, and the first real pyramid, the one in Meidum, was built with Khufu's slope of 22:1. The next pyramid, the Bent Pyramid in Dahshur, was begun in a steeper 21:1 slope, but this was lowered in the upper third, probably because a setting of the foundation[9 ]. All later pyramids up to the 12th dynasty were built with slopes between 22:1 and 20:1, with a clustering of 21:1 and 20:1 slopes.
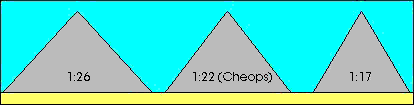
Here you see three pyramid slopes. In my opinion the 22:1 looks most aesthetic and dynamic. Slopes steeper than 20:1 were probably impossible in large buildings, and the shallow buildings look weak and tired. The most aesthetic pyramid in Giza is for example the steep one of Kafre.
I think it is now clear, that there is no planned π-coding in the Great pyramid, and that this value is only an artefact of the measurement system the Egyptians used. Therefore mysteries (7a), (8a) and (12) are out of the race. Where π is not codet into, no π-formulas can b obtained from.
Already 7 of the original 19 mysteries have been disproved. It gets narrower...
Especially because two more riddles can be solved en passant:: Riddle (8b) should be a “monument for squaring the circle”. This means the geometrical (impossible) construction of a square with the same area than a given circle only with rulers and compasses. And with that the Great Pyramid really has nothing to do.
Riddle (14), the representation of Earth by the pyramid, can be disproved with the same values von Däniken gives. The circumference of the pyramid with 4 x 230,38 meters should represent earth's circumference of 40076.592 kilometres, whereas the pyramid's height should represent with the formula “2 π x height” the polar circumference of 40009.153 km. Both circumferences differ about 67 kilometres. Using the values of von Däniken we get:
Again an assertion von Däniken copied without checking. Or trusting, that his readers never do.
By the way: the values von Däniken gives are hopelessly outdated. Modern literature gives the equatorial radius with 6378.140 km (the circumference is then 40075 km) and the polar radius with 6356.775 km (that gives a circumference of 39941 km)[10 ]. The difference between these two circumferences is with 134 km twice as large as with the values of von Däniken. Just a coincidence? :-)
There exists another form of this “riddle”. According to that, the pyramid “is a scale model of the northern hemisphere in the scale of 1:43200“[11 ]. If we draw a circle around the pyramid (either an inner circle touching tha pyramid sides, or an outer circle with the pyramid edges touching it – different authors give different concepts) the height again gives the polar radius. This we can also check with pushing a few keys on a pocket calculator. Both concepts work with radius ratios, the equatorial radius is given either by the half base length or the half diagonal. From the data given above the ratio between the equatorial radius and the polar radius of earth is 1.00336. Let's see what these pyramid models give us:
Both concepts don't work. Now we have eliminated 9 pyramid “mysteries”, 10 more have to be checked..
| Remarks: | |
| [1 ] | Taylor, John; The great Pyramid, who built it and why, London 1854 |
| [2 ] | Däniken; Sphinx, p. 155 |
| [3 ] | Lehmann, Johannes; So rechneten Ägypter und Babylonier, Urania 1994, p. 48 f |
| [4 ] | Gillings, Richard J.; Mathematics in the time of the Pharaos, MIT Press 1972, p. 139 ff |
| [5 ] | ibd. S. 140 |
| [6 ] | Conceived by electronics engineer T.E.Conelly, presented to a larger audience in the book Riddles of the pyramids by Kurt Mendelssohn, and presented in Germany to millions of TV viewers in the science-magazine “Querschnitte” by presenter Hoimar von Dithfurt. |
| [7 ] | All examples can be found in original writing and translation in Lehmann. |
| [8 ] | The wall rings of the step pyramids are much steeper than the pyramid shape, more than 70°. But if one constructs a hull covering the edges of the steps (like the Egyptians did it in Meidum) we get this range of angles. | [9 ] | There are hypotheses, that the pyramid was planned this way, as a double pyramid. Pros are the seperate entries (west and north, the two separate chamber systems and the carefully finishing. Also, this pyramid is the best preserved one in the whole of Egypt.. | [10 ] | Lang/Whitney; Planets, p. 370 |
| [11 ] | z.B in Hancock, Graham; Fingerprints of the Gods, p. 178 ff |